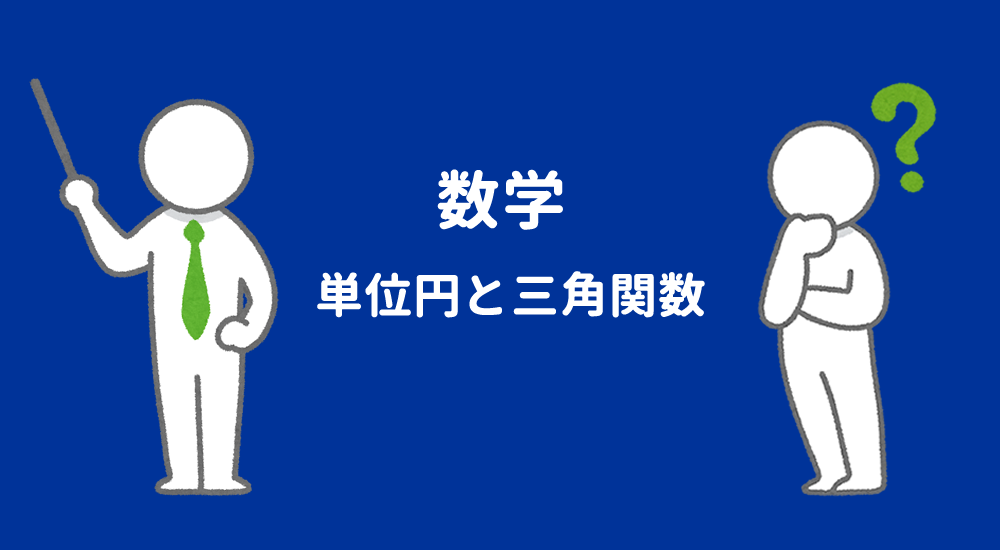
単位円の円周上に点$P$をとるとき、その座標を$(1, \, 0 )$からの角度$\theta$を用いて、$P$の座標を$(\cos\theta, \, \sin\theta )$とします。
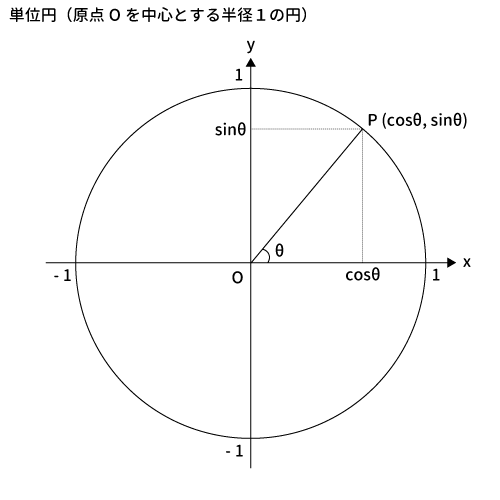
この$P$と原点$O$などから出来る三角形を転がして三角関数の関係式をいろいろとチェックしたいと思います。覚える負担を少しでも減らすことができれば幸いです。
転がす三角形
点$P$から$x$軸に下ろした垂線と$x$軸との交点を$A$とします。このとき、単位円の円周上の点$P$、原点$O$、点$A$の3点でできる$\bigtriangleup OAP$を転がして三角関数の関係を見ていきたいと思います。
この三角形は原点$O$を中心に回転させていくと、$OP$部分は常に$1$($P$は常に単位円の円周上)なので各関係性を見つけやすいです。
各辺の長さ
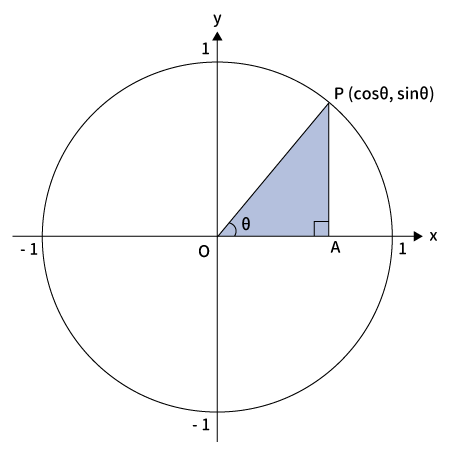
$P$の座標$(\cos\theta, \, \sin\theta )$より、
$OA=\cos\theta$
$AP=\sin\theta$
単位円より、半径$1$なので、
$OP=1$
$OA=\cos\theta$
$AP=\sin\theta$
$OP=1$
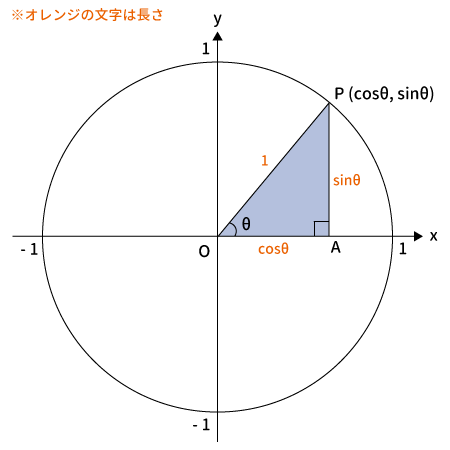
$\tan \theta$
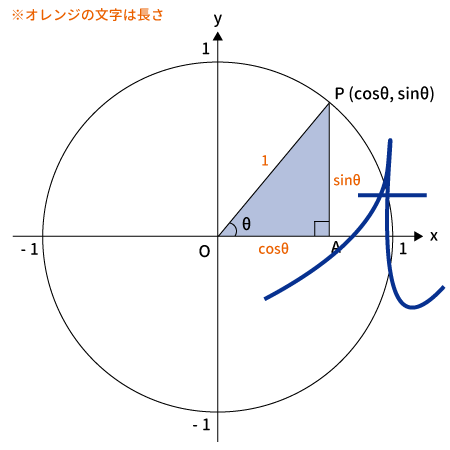
$\tan \theta$は定義より、上の画像の通りなので
$\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$
得られる関係式
$con^2 \theta + \sin^2 \theta=1$
$\cos(90 ^{\circ}+\theta)=-\sin\theta$
$\sin(90 ^{\circ}+\theta)=\cos\theta$
$\displaystyle \tan(90 ^{\circ}+\theta) = -\frac{1}{\tan\theta}$
$\cos(180 ^{\circ}+\theta)=-\cos\theta$
$\sin(180 ^{\circ}+\theta)=-\sin\theta$
$\tan(180 ^{\circ}+\theta) = \tan \theta$
$\cos(270 ^{\circ}+\theta)=\sin\theta$
$\sin(270 ^{\circ}+\theta)=-\cos\theta$
$\displaystyle \tan(270 ^{\circ}+\theta) = -\frac{1}{\tan\theta}$
では、一つずつ見ていきましょう。
三平方の定理より
転がす前に三平方の定理より関係式が得られますので、そちらから確認しておきます。

三平方の定理より、$OP^2=OA^2+AP^2$なので、
$OP^2=con^2 \theta + \sin^2 \theta$
$OP=1$なので、$OP^2=1^2=1$となり、
$con^2 \theta + \sin^2 \theta=1$
という関係式が得られました。
$con^2 \theta + \sin^2 \theta=1$
詳細は「【三角比の相互関係】$\cos^2 \theta + \sin^2 \theta=1$は単位円で簡単に理解できる」をご覧ください。
$90 ^{\circ}$回転($90 ^{\circ}+\theta$)
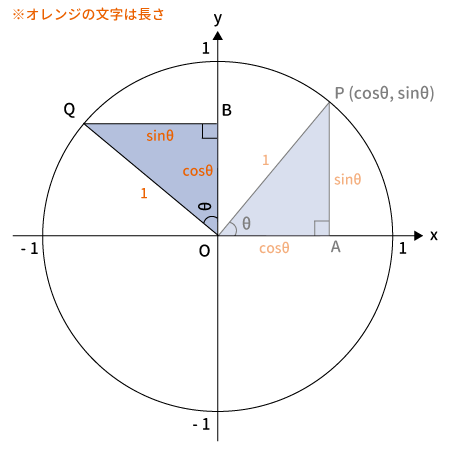
上図のように$\bigtriangleup OAP$を$90 ^{\circ}$回転させた後の点を$Q$、$B$とします。
Qの座標
$Q$は$\theta$を$90 ^{\circ}$回転させたものなので、$Q$の座標は$(\cos(90 ^{\circ}+\theta), \, \sin(90 ^{\circ}+\theta))$です。
※$x$座標は$\cos$、$y$座標は$\sin$となります。詳細は「単位円とは?」をご覧ください
また、$\displaystyle \tan (90 ^{\circ}+\theta) = \frac{\sin (90 ^{\circ}+\theta)}{\cos (90 ^{\circ}+\theta)}$です。

$\bigtriangleup OBQ$の各辺の長さ
回転させても三角形の各辺の長さは変わらないので、下記が成り立ちます。
$OB=\cos\theta$
$BQ=\sin\theta$
$OQ=1$
各辺の長さからも$Q$の座標を求める
$\bigtriangleup OBQ$の各辺の長さからも$Q$の座標を求めることができます。
下図を見てわかる通り、$Q$の$x$座標は$-BQ$、$y$座標は$OB$なので、$Q$の座標は$(-\sin\theta, \, \cos\theta)$となります。

$Q$の座標$(\cos(90 ^{\circ}+\theta), \, \sin(90 ^{\circ}+\theta))=(-\sin\theta, \, \cos\theta)$
上記より$Q$の座標は$(\cos(90 ^{\circ}+\theta), \, \sin(90 ^{\circ}+\theta))$と$(-\sin\theta, \, \cos\theta)$と2通りの方法で表せたので、$x$、$y$座標同士がそれぞれ等しいということになります。
よって、
$\cos(90 ^{\circ}+\theta)=-\sin\theta$
$\sin(90 ^{\circ}+\theta)=\cos\theta$
$\tan (90 ^{\circ}+\theta)$
$\displaystyle \tan (90 ^{\circ}+\theta) = \frac{\sin (90 ^{\circ}+\theta)}{\cos (90 ^{\circ}+\theta)}$なので、上記2つを代入すると、
$\displaystyle \tan(90 ^{\circ}+\theta) = \frac{\cos\theta}{-\sin\theta}$
さらに、$\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$より、$\displaystyle \frac{\cos \theta}{\sin \theta} = \frac{1}{\tan\theta}$。これを代入すると、
$\displaystyle \tan(90 ^{\circ}+\theta) = -\frac{1}{\tan\theta}$
$90 ^{\circ}+\theta$と$\theta$の関係式
$\cos(90 ^{\circ}+\theta)=-\sin\theta$
$\sin(90 ^{\circ}+\theta)=\cos\theta$
$\displaystyle \tan(90 ^{\circ}+\theta) = -\frac{1}{\tan\theta}$
$180 ^{\circ}$回転($180 ^{\circ}+\theta$)
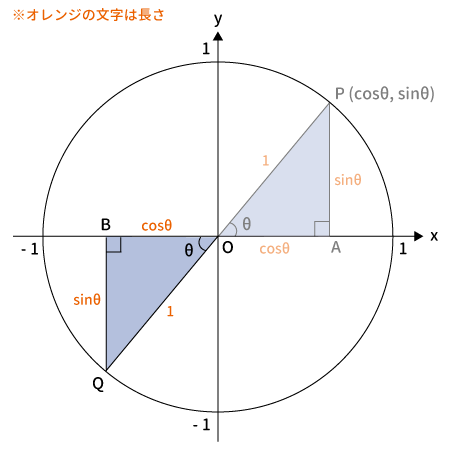
上図のように$\bigtriangleup OAP$を$180 ^{\circ}$回転させた後の点を$Q$、$B$とします。
Qの座標
$Q$は$\theta$を$180 ^{\circ}$回転させたものなので、$Q$の座標は$(\cos(180 ^{\circ}+\theta), \, \sin(180 ^{\circ}+\theta))$です。
※$x$座標は$\cos$、$y$座標は$\sin$となります。詳細は「単位円とは?」をご覧ください
また、$\displaystyle \tan (180 ^{\circ}+\theta) = \frac{\sin (180 ^{\circ}+\theta)}{\cos (180 ^{\circ}+\theta)}$です。

$\bigtriangleup OBQ$の各辺の長さ
回転させても三角形の各辺の長さは変わらないので、下記が成り立ちます。
$OB=\cos\theta$
$BQ=\sin\theta$
$OQ=1$
各辺の長さからも$Q$の座標を求める
$\bigtriangleup OBQ$の各辺の長さからも$Q$の座標を求めることができます。
下図を見てわかる通り、$Q$の$x$座標は$-OB$、$y$座標は$-BQ$なので、$Q$の座標は$(-\cos\theta, \, -\sin\theta)$となります。
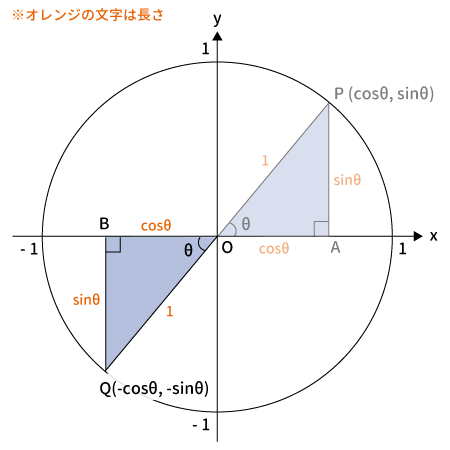
$Q$の座標$(\cos(180 ^{\circ}+\theta), \, \sin(180 ^{\circ}+\theta))=(-\cos\theta, \, -\sin\theta)$
上記より$Q$の座標は$(\cos(180 ^{\circ}+\theta), \, \sin(180 ^{\circ}+\theta))$と$(-\cos\theta, \, -\sin\theta)$と2通りの方法で表せたので、$x$、$y$座標同士がそれぞれ等しいということになります。
よって、
$\cos(180 ^{\circ}+\theta)=-\cos\theta$
$\sin(180 ^{\circ}+\theta)=-\sin\theta$
$\tan (180 ^{\circ}+\theta)$
$\displaystyle \tan (180 ^{\circ}+\theta) = \frac{\sin (180 ^{\circ}+\theta)}{\cos (180 ^{\circ}+\theta)}$なので、上記2つを代入すると、
$\displaystyle \tan(180 ^{\circ}+\theta) = \frac{-\sin\theta}{-\cos\theta}=\frac{\sin\theta}{\cos\theta}$
さらに、$\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$を代入すると、
$\tan(180 ^{\circ}+\theta) = \tan \theta$
$180 ^{\circ}+\theta$と$\theta$の関係式
$\cos(180 ^{\circ}+\theta)=-\cos\theta$
$\sin(180 ^{\circ}+\theta)=-\sin\theta$
$\tan(180 ^{\circ}+\theta) = \tan \theta$
$270 ^{\circ}$回転($270 ^{\circ}+\theta$)

上図のように$\bigtriangleup OAP$を$270 ^{\circ}$回転させた後の点を$Q$、$B$とします。
Qの座標
$Q$は$\theta$を$270 ^{\circ}$回転させたものなので、$Q$の座標は$(\cos(270 ^{\circ}+\theta), \, \sin(270 ^{\circ}+\theta))$です。
※$x$座標は$\cos$、$y$座標は$\sin$となります。詳細は「単位円とは?」をご覧ください
また、$\displaystyle \tan (270 ^{\circ}+\theta) = \frac{\sin (270 ^{\circ}+\theta)}{\cos (270 ^{\circ}+\theta)}$です。
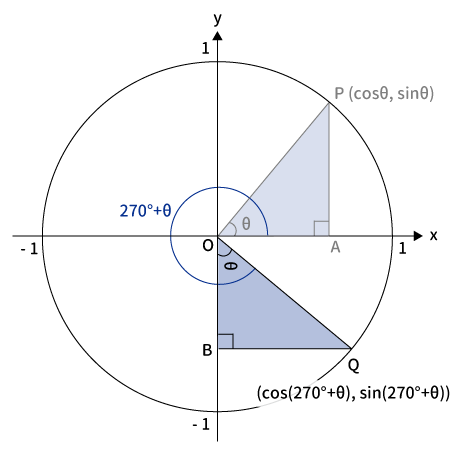
$\bigtriangleup OBQ$の各辺の長さ
回転させても三角形の各辺の長さは変わらないので、下記が成り立ちます。
$OB=\cos\theta$
$BQ=\sin\theta$
$OQ=1$
各辺の長さからも$Q$の座標を求める
$\bigtriangleup OBQ$の各辺の長さからも$Q$の座標を求めることができます。
下図を見てわかる通り、$Q$の$x$座標は$BQ$、$y$座標は$-OB$なので、$Q$の座標は$(\sin\theta, \, -\cos\theta)$となります。
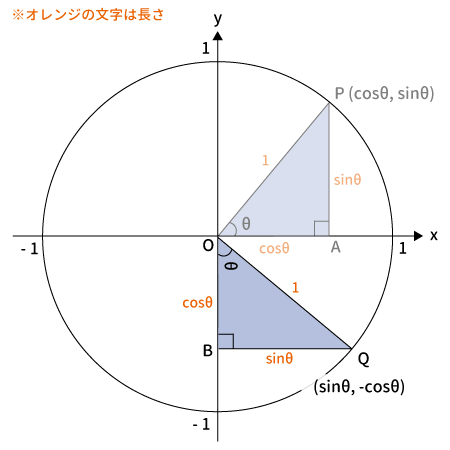
$Q$の座標$(\cos(270 ^{\circ}+\theta), \, \sin(270 ^{\circ}+\theta))=(\sin\theta, \, -\cos\theta)$
上記より$Q$の座標は$(\cos(270 ^{\circ}+\theta), \, \sin(270 ^{\circ}+\theta))$と$(\sin\theta, \, -\cos\theta)$と2通りの方法で表せたので、$x$、$y$座標同士がそれぞれ等しいということになります。
よって、
$\cos(270 ^{\circ}+\theta)=\sin\theta$
$\sin(270 ^{\circ}+\theta)=-\cos\theta$
$\tan (270 ^{\circ}+\theta)$
$\displaystyle \tan (270 ^{\circ}+\theta) = \frac{\sin (270 ^{\circ}+\theta)}{\cos (270 ^{\circ}+\theta)}$なので、上記2つを代入すると、
$\displaystyle \tan(270 ^{\circ}+\theta) = \frac{-\cos\theta}{\sin\theta}$
さらに、$\displaystyle \tan \theta = \frac{\sin \theta}{\cos \theta}$より、$\displaystyle \frac{\cos \theta}{\sin \theta} = \frac{1}{\tan\theta}$。これを代入すると、
$\displaystyle \tan(270 ^{\circ}+\theta) = -\frac{1}{\tan\theta}$
$270 ^{\circ}+\theta$と$\theta$の関係式
$\cos(270 ^{\circ}+\theta)=\sin\theta$
$\sin(270 ^{\circ}+\theta)=-\cos\theta$
$\displaystyle \tan(270 ^{\circ}+\theta) = -\frac{1}{\tan\theta}$
スポンサーリンク
- 問題の解答例など解法は見ずに解いています。覚えていない部分は学習はしますが、直接問題の解き方は調べていません。
よって、順当な解法かは分かりませんが、何か参考になれば幸いです - なるべく細かく書くようにしています。不明点はコメントいただければ嬉しいです
- また、正解かどうかのチェックは行い、正解にはなっています
キーワード
気になる人は調べてみてね。
三角関数、単位円、三平方の定理、$\sin \theta$、$\cos \theta$、$\tan \theta$